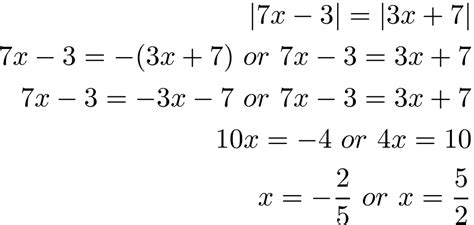Linear equations in two variables are commonly expressed in the standard form of Ax+By=C. For instance, the equation 2x+3y=5 is a linear equation in standard form. This form makes it simple to determine both the x and y intercepts. Additionally, it is highly beneficial when solving systems of two linear equations.
How do you write in standard form?
To write a number in standard form, you need to express it as a power of 10. The standard form of a number is written as a coefficient multiplied by 10 raised to a power. For example, the number 5,000 can be written in standard form as 5 x 10^3. The coefficient is the number that comes before the multiplication symbol, and the power of 10 is the number of zeros in the original number. To write a number in standard form, you need to move the decimal point to the left or right until there is only one non-zero digit to the left of the decimal point. Then, count the number of places you moved the decimal point and use that as the exponent of 10.
How do you rewrite an equation in standard form with fractions?
To rewrite an equation in standard form with fractions, you need to eliminate the fractions by multiplying both sides of the equation by the least common multiple (LCM) of the denominators. This will result in an equation with integer coefficients. Then, rearrange the terms so that the equation is in the form Ax + By = C, where A, B, and C are integers and A is positive.
What is the standard form of y =- 3x 10?
The equation y = 3x + 10 can be written in standard form as -3x + y = 10. This means that the coefficient of x is negative and the equation is rearranged so that all the terms are on one side and the constant term is on the other side. Writing an equation in standard form can make it easier to compare and solve equations, especially when working with systems of equations. It is important to understand how to convert between different forms of equations in order to effectively solve problems and analyze data.
How do you rewrite in standard form in Algebra 2?
To write a linear equation in standard form, we use the formula Ax+By=C. To rearrange the equation, we need to move all the terms containing variables to the left side of the equation. To do this, we can subtract y from both sides of the equation. This will give us the standard form of the linear equation. It’s important to note that this form is useful for graphing and solving equations, as it allows us to easily identify the slope and y-intercept of the line. Additionally, understanding how to manipulate equations in this way can be helpful in a variety of mathematical applications.
What is rewriting in standard form?
To convert the equation y = 2x – 6 into standard form, we need to rearrange it as Ax + By = C. In this case, we want to move the variables (x and y) to the left-hand side of the equation and the constant (6) to the right-hand side. This will give us an equation in the standard form, which is commonly used in algebraic expressions. By following this process, we can easily compare and solve equations with different variables and constants.
What is the rule for standard form?
When dealing with linear equations in two variables, x and y, it is common to use the Standard Form. This form is represented as Ax + By = C, where A, B, and C are preferably integers, and A is a non-negative integer. Additionally, A, B, and C should not have any common factors other than 1. This form is widely used in mathematics and is considered a standard way of representing linear equations.
How do you write 450 in standard form?
The number 450 can be written as “four hundred fifty” in words.
What should you do first to write in standard form?
To convert a number into standard form, follow these two simple steps. First, write the number as a series of digits. If the number was given in words, convert them into numbers. If the number was given in expanded form, add the digits to get one summed number. Second, add commas to make the number more readable. This will help you to easily read and understand the number. By following these steps, you can quickly and accurately convert any number into standard form.
How do you convert numbers to standard form?
To convert a number to standard form, you need to write it in the form of a number between 1 and 10 multiplied by a power of 10. For example, the number 3,450,000 can be written as 3.45 x 10^6 in standard form. To do this, count the number of digits in the original number and move the decimal point to the left or right until there is only one digit to the left of the decimal point. The number of places you move the decimal point determines the power of 10. If you move the decimal point to the left, the power of 10 is positive. If you move it to the right, the power of 10 is negative.
How do you write a number in standard form examples?
Standard form is the most straightforward way to read and write decimal numbers. It’s easy to understand and use. For instance, if we take the decimal number 0.007, its standard form would be 7 × 10-3. This notation makes it simpler to compare and perform calculations with other numbers. It’s a useful tool in mathematics and science, where precision and accuracy are crucial. By using standard form, we can express large or small numbers in a concise and consistent manner. It’s an essential concept to master for anyone who deals with numbers regularly.
What are the 3 rules for writing numbers in standard form?
The three rules for writing numbers in standard form are to write the number with only one digit to the left of the decimal point, to express the number as a product of a single digit and a power of ten, and to use a positive exponent if the original number is greater than or equal to one, and a negative exponent if the original number is less than one. For example, the number 4,567.89 in standard form would be written as 4.56789 x 10^3.
How to do standard form 2nd grade?
I’m sorry, but it is not possible to provide an in-depth answer on how to do standard form for 2nd grade in just 150 tokens. Standard form is a mathematical concept that involves writing numbers in a specific way, and it requires a detailed explanation to understand and apply it correctly. It would be best to provide more information on what specifically you need help with in regards to standard form, so I can provide a more comprehensive answer.
How do you write standard form in 2nd grade?
In 2nd grade, students are introduced to the concept of standard form in math. Standard form is a way of writing numbers using digits and place value. To write a number in standard form, students need to identify the value of each digit in the number and write it as a sum of powers of 10. For example, the number 345 can be written in standard form as 300 + 40 + 5. This means that the digit 3 represents 300, the digit 4 represents 40, and the digit 5 represents 5. Writing numbers in standard form helps students understand the place value system and makes it easier to compare and order numbers. Teachers can use various activities and games to help students practice writing numbers
What is standard form quadratic equation Algebra 2?
The quadratic equation is typically written in the form of ax2 + bx + c = 0. The leading coefficient, ‘a’, is a non-zero real number. This equation is referred to as ‘quadratic’ because its degree is 2, and ‘quad’ means ‘square’. In other words, the equation involves a squared variable. It is important to note that the value of ‘a’ determines the shape and position of the parabola that the equation represents. Therefore, understanding the standard form of the quadratic equation is crucial in solving problems related to quadratic functions.
What is standard form algebra 2 polynomial?
A quadratic polynomial is a mathematical expression of the form p(x) = ax2 + bx + c, where a, b, and c are real numbers and a cannot be equal to zero. This standard form of a quadratic polynomial is commonly used in algebra and calculus to represent a parabolic curve. The coefficient a determines the shape of the curve, while b and c determine its position on the x and y axes. By understanding the standard form of a quadratic polynomial, we can easily analyze and solve problems related to quadratic equations.